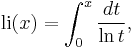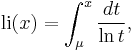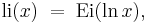Ramanujan–Soldner constant

In mathematics, the Ramanujan–Soldner constant (also called the Soldner constant) is a mathematical constant defined as the unique positive zero of the logarithmic integral function. It is named after Srinivasa Ramanujan and Johann Georg von Soldner.

Its value is approximately μ ≈ 1.451369234883381050283968485892027449493… (sequence A070769 in OEIS)

Since the logarithmic integral is defined by

\mathrm{li}(x) = \int_0^x \frac{dt}{\ln t},

we have

\mathrm{li}(x)\;=\;\mathrm{li}(x) - \mathrm{li}(\mu)
\int_0^x \frac{dt}{\ln t} = \int_0^x \frac{dt}{\ln t} - \int_0^{\mu} \frac{dt}{\ln t}
\mathrm{li}(x) = \int_{\mu}^x \frac{dt}{\ln t},

thus easing calculation for positive integers. Also, since the exponential integral function satisfies the equation

\mathrm{li}(x)\;=\;\mathrm{Ei}(\ln{x}),

the only positive zero of the exponential integral occurs at the natural logarithm of the Ramanujan–Soldner constant, whose value is approximately ln(μ) ≈ 0.372507410781366634461991866… (sequence A091723 in OEIS)

External links